Quasi-Geostrophic Turbulence

Abstract

Large parts of the Earth's Atmosphere and Ocean have a gravitationally stable density stratification, with lighter fluid above heavier fluid. This stable stratification, combined with the planetary rotation, causes the most energetic motion to occur approximately in horizontal planes. For this situation we solve an approximate set of equations, called the Quasi-Geostrophic equations, using a fully implicit multigrid algorithm. In solutions for the decay from random initial conditions, we see the spontaneous emergence of concentrated vortices that regulate the evolution of the flow. The inclusion of the "beta effect", i.e., the variation in the Coriolis force with latitude due the Earth's sphericity, introduces Rossby waves and jets which compete with the vortices.

Project Description

The discovery of "coherent structures" in turbulent fluid flow has certainly been one of the most prominent advances in the field of fluid mechanics in the last 25 years. A "coherent structure" may be thought of as a shape or form in a turbulent fluid flow that persists a long time relative to it's own period of internal circulation. One example of a "coherent structure" is the Great Red Spot of Jupiter's atmosphere, a long-term stable pattern in the chaotic and energetic flow of the Jovian atmosphere.

The primary goal of this project is to calculate fluid turbulence under the influences of environmental rotation and variable density at unprecedentedly high resolution by using the largest, hence newest, available computers. The guiding hypothesis is that this will provide a superior depiction of fully developed turbulence and thus provide new insights into its fundamental nature.

As part of this project, new codes have been developed to calculate fluid motions under the approximation of incompressibility. This is appropriate for slow motions (with small Mach number), such as those that occur on large scales in the earth's atmosphere and ocean, as well as in laboratory fluid tanks. These codes are currently being run on the Cray C-90 computer at the Pittsburgh Supercomputing Center, and are being ported to the Cray T3D.

I. f-Plane

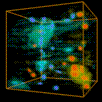
Below are images of the potential vorticity field for a computation on an "f-plane", i.e. rotation with constant Coriolis parameter, at a resolution of 320^3. One sees that starting from random initial conditions, the fluid self-organizes into coherent vortices. These vortices subsequently advect each other, merge to form larger vortices, and align vertically.

Still Images

Animations

Another web page about these computations can be found at NCAR.

II. Beta-Plane

Below are images of the potential vorticity field from a simulation on the "beta-plane", i.e. with linear latitudinal variation in the Coriolis parameter, at a resolution of 256^3. The coherent vortices now grow through merger and alignment until they reach the scale where the beta-effect becomes important, at which point dispersive Rossby waves are excited, destroying the vortices and producing horizontal jets.
References